Failures of the classical optical theorem under arbitrary-shaped beam incidence in electromagnetism, acoustics, and quantum mechanics: motivation and a review

1 Introduction

The classical optical theorem states that for an electromagnetic wave propagating in a lossless medium and incident on a finite scatterer, the extinction cross section (total cross section for elastic plus inelastic scattering), either for a spherical or non-spherical particle, is proportional to the real part of the scattering amplitude in the forward direction [1–3]. This optical theorem should not be confused with the Ewald–Oseen extinction theorem, sometimes also known as an optical theorem, which states that a wave incident at the speed of light on a medium of different refractive indexes is extinguished by light emitted by the atom at the speed of light and is replaced by a wave (the refracted wave) traveling at a speed slower than the speed of light [4].

The story of the electromagnetic optical theorem that will be discussed in the present paper began in 1982, at the earlier stage of development of what is now known as the generalized Lorenz–Mie theory. This theory describes the interaction between an illuminating electromagnetic arbitrary-shaped beam (or structured beam, typically a laser beam) and a homogeneous sphere defined by its radius and its complex refractive index of refraction, as shown in [5] and references therein. In the most general case, the incident beam is encoded by two sets of beam shape coefficients (BSCs) which are usually denoted as $g_{n,TM}^m$ and $g_{n,TE}^m$ (n from 1 to ∞, m from (−n) to (+n), TM for “transverse magnetic,” and TE for “transverse electric”). In some cases, in particular for on-axis Gaussian beams, e.g., [6], this double set of coefficients may be reduced to a single set of uni-index BSCs g_n in which the distinction between transverse magnetic and transverse electric waves is washed out. The first archival paper on GLMT, dated 1982 [7], was restricted to such a special framework, using the uni-index BSCs for the first time, which, nevertheless, has been sufficient to demonstrate, soon after, the failure of the electromagnetic optical theorem. Although not released in the archival literature, the demonstration of this failure has been stored in internal reports issued in 1984 [8, 9]. This has been followed by a long story before the discovery in 2014, published in 2016 [10], of the deep reason for failure. In this story, there has been two surprises: 1) the failure of the optical theorem was completely unexpected and, in the recent words of Markel [11], “it came as a bit of surprise that the optical theorem (in its conventional form) does not hold for sufficiently narrow Gaussian beams”; 2) although several analytical works have been performed to study this failure, it is only approximately three decades after its discovery that the gist of it has been understood and could be explained without the requirement of any equation [10].

This constitutes what may be called a “wow” story which is discussed in Section 2 of the present paper. The contents of this paper are afterward complemented as follows. Section 3 provides a discussion of worldwide contributions to the electromagnetic optical theorem. There also exist other versions of the optical theorem, in acoustics and in quantum mechanics. The case of acoustical waves is discussed in Section 4, while quantum mechanics is discussed in Section 5. Finally, Section 6 provides the conclusion.

2 The wow story of the electromagnetic optical theorem

For the expression of the extinction cross section C_ext, the reader may most conveniently refer to Eq. 6.58 in [5] to obtain

in which λ is the wavenumber in the free space surrounding the scatterer, a_n and b_n are the Mie coefficients encoding the properties of the scatterer, and g_n denotes the uni-index BSCs. Figure 1 shows the 1984-vintage version of the same expression.

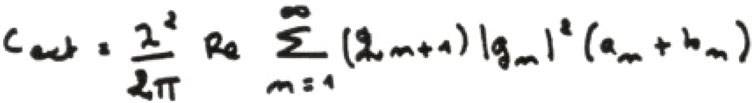
FIGURE 1. Vintage version of Eq. 1 from a 1984 internal report.

Equation 1 has been published for the first time in the archival literature in 1985 [12]. For convenience, when dealing with the sequel, Eq. 1 is better rewritten as follows:

We may also directly use the optical theorem, which tells us that C_ext is given by [1–3]

where S(0) is the light-scattering amplitude in the forward direction for θ = 0, given by [13]

where in the uni-index GLMT framework [7],

where π_n and τ_n denote the generalized Legendre functions.

where $P_n^m$ is an associated Legendre function, expressed using Hobson’s definition according to [14].

where P_n denotes the usual Legendre polynomials.

In the forward direction θ = 0 (cos θ = 1), the generalized Legendre functions to be used, with Hobson’s notation, are found to be

so Eqs 3–6 lead to

For a plane wave with parallel illumination (for oblique illumination, see [15]), which is the usual Mie configuration, the uni-index BSCs are constant phase factors, which are usually just taken equal to 1, e.g., Section 6.4 in [5]. Then, Eqs 2, 11 identify as they should. However, in general, they are different, illustrating the failure of the classical theorem for arbitrary-shaped beams. Another example is provided by a beam known as the top-hat beam, which may be used for optical particle sizing (OPS) [16–18] and whose uni-index BSCs are g_n = 1 for 1 ≤ n ≤ n_max and g_n = 0 for n > n_max [19]. This beam, although exhibiting transverse localization, behaves as a plane wave in both amplitude and phase in its plateau region.

The results associated with Eqs 1–11 were not sufficient enough to be published in the archival literature. Furthermore, the emergency was to complete the GLMT formalism and to make it useable for practical applications. Due to algorithmic difficulties associated with excessive computational times and keeping in mind applications in the field of OPS requiring successful computations for large enough particles (say with a diameter of approximately 100 μm), the first relevant computations have been published only in 1988 by Corbin et al. [20], and the first genuine applications to OPS have been published only in 1993–1994 by Gréhan et al. [21–23].

This was then the right time to return to the optical theorem issue. The optical theorem material described previously has then been incorporated in a paper published in 1995 by Lock et al. [24]. Then, let us consider the case of an on-axis Gaussian beam, propagating in the z-direction, polarized in the x-direction at the waist, and described using what is known as a localized approximation in which the uni-index BSCs read as follows [25–29]:

where s is the beam’s confinement factor, which is defined as

where w₀ is the beam’s waist radius. The value of s ranges from 0 for a plane wave (corresponding to w₀ → ∞) to s ∼ 1/(2π) ∼ 0.16 when the beam is focused down to the diffraction limit with w₀ ∼ λ. For a plane wave, we obtained g_n = 1 so that the classical optical theorem is indeed valid.

It should be noted that the uni-index BSCs in Eq. 12 are real numbers, and Taylor expanding one of the BSCs (but not both of them) in Eq. 1, we obtain

where

We then remark that the j = 0 term in Eq. 14 reads as follows:

which is exactly the result of Eq. 11, now rewritten as C_ext(OT) obtained by using the classical optical theorem, and does not depend on the beam confinement factor. Therefore, Eq. 14 may be rewritten as follows:

which shows that C_ext is equal to the extinction cross section given by the classical optical theorem, supplemented by an infinite series in terms of successive powers of s². The paper proposed by Lock et al. [24] is complemented by studying two special cases, i.e., the wide-beam approximation when w₀ ≥ a (in which a is the radius of the spherical scatterer) and the narrow-beam approximation when w₀ ≤ a.

The wide-beam approximation is studied using two assumptions 1) that diffraction dominates all other scattering processes in the forward direction, allowing one to use a Debye-series decomposition of the partial-wave scattering amplitude and 2) that the plane-wave extinction efficiency is roughly equal to 2, e.g., [30], pp. 120–122. We then obtain

where the expression for the classical optical theorem is complemented by an O(s²)-term. Since the wide-beam approximation “approaches” the case of a plane wave, only one correction O(s²)-term is required. Again, for a plane wave, i.e., s → 0, Eq. 18 reduces to Eq. 3. The result for the narrow–wide approximation is more involved and, therefore, not reproduced here.

The same issue, namely, the generalized optical theorem for on-axis Gaussian beams, was considered in 1996 by Gouesbet et al. [31]. The uni-index BSCs are given as follows:

where three different approaches are considered. In the first approach, N_l = n_l, where

A beam described by Eq. 19 is called a standard beam. It is obtained by generalizing to infinite order the expressions of the BSCs obtained when dealing with the first-order, third-order, and fifth-order Davis scheme of approximations [6, 19, 32]. However, let us note that the standard scheme has a finite radius of convergence and has afterward been modified to an improved standard scheme [33]. Furthermore, it has unexpectedly been demonstrated that the Davis scheme of approximations, the standard scheme, and the improved standard scheme all lead to asymptotic series, similar to the ones encountered in quantum electrodynamics, that is to say, series whose first terms provide a satisfactory answer to the problem in hand, although they eventually diverge [34].

In the second approach,

which simply corresponds to the localized approximation of Eq. 12, while the third approach leads to

which corresponds to an improved localized approximation. For the improved localized approximation and variants, see [35–38] for a review and [39–41] for complements.

Inserting Eq. 19 into Eq. 2 leads to

where

where the first term in the series of Eq. 24, with pw standing for the “plane wave,” is expressed in terms of the optical theorem, i.e., using Eq. 11 with g_n = 1 as follows:

This is in contrast with Eq. 17 in which the first term C_ext(OT) given by Eq. 11 is obtained by applying the optical theorem to the focused beam, in which the BSCs g_n are the ones of the shaped beam and not the ones for a plane wave. However, in both cases, the first term is complemented by a series in powers of s². It should also be noted that in the case of a standard beam with N_l given by n_l in Eqs 20, 21, we expect that Eq. 24 would generate an asymptotic series.

Furthermore, for the localized approximation and modified localized approximation cases, we may use

and establish that Eq. 25 reduces to

where X = (n + 1/2)² in the localized approximation case and (n − 1)(n + 2) in the modified localized approximation. Finally, we may also establish [31]

in which the first term now corresponds to the classical optical theorem applied to the shaped beam and F_2l is given by

which is observed to be similar to Eq. 25, but for the summation index i which starts from 1 instead of 0.

At this stage, we possess a fairly complete analytical discussion of the failure of the optical theorem, together with additional comments and numerical results obtained from [24, 31]. The issue has then been put aside for another 10 years. The next ingredient for the final step has been the introduction of the Angular Spectrum Representation (ASR). Instead of encoding the shaped beam by using BSCs, it is indeed possible to represent it as a combination of incident tilted plane waves. This ASR has been extremely undervalued for a long time because, when handled numerically, it performs very poorly as exemplified by a paper from Lock [42], see also pp. 52–54 in [5]. However, we now know that ASR may be in some cases handled analytically that it may then be used to evaluate the BSCs of GLMT and then provides a new method to complement the arsenal of methods which can be used to encode the shaped beams, see, for instance, recent works in [43–45], [46], [47], [48] and the review in [49].

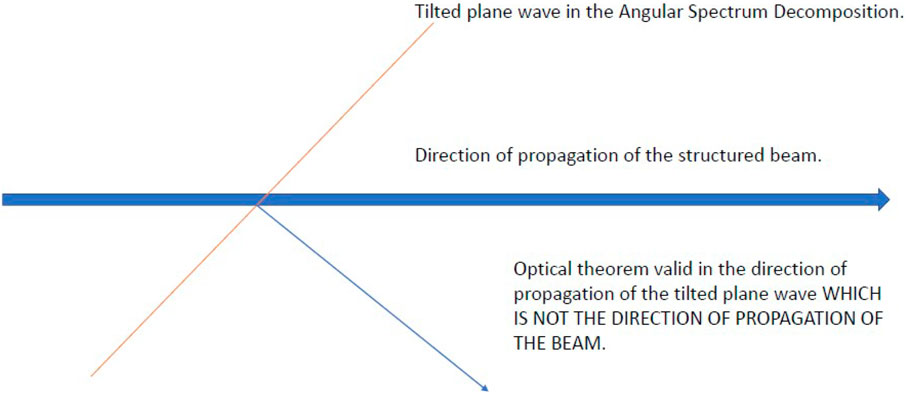
In the framework of the present story, two papers published in 2015 by Gouesbet and Lock are important [50, 51]. It means that, in 2014, the two authors of these papers had everything in mind for the final step. This final step happened in September 2014, during a tour in the French Alps, after the “laser-light and interaction with particles” conference held in Marseille from 25 to 29 August [52]. During a meal, Lock began a sentence which was completed by Gouesbet. The content of this sentence is explained in Figure 2, which provides the final explanation of the failure of the optical theorem for shaped beams: The classical optical theorem applies with respect to the direction of propagation of each tilted plane wave of ASR, but these directions generically do not identify with the direction of the beam. In other words, the plane-wave illumination assumption behind the optical theorem is very restrictive, i.e., the plane-wave illumination assumption is a prerequisite for the application of the optical theorem, as explicitly stated, for example, in [53–55]. This will also apply to acoustical and quantum mechanical waves discussed in the sequel.

FIGURE 2. Understanding the failure of the optical theorem.

This is the end of the wow story for the optical theorem, but it must be complemented by an additional wow result which is strongly connected to the optical theorem issue by an incredible coincidence. Soon after the revelation of the deep reason as to why the classical optical theorem fails for structured beams, one of them (G. Gouesbet) heard of an experimental result, according to which photons in a structured beam travel at a velocity slower than the speed of light (even in vacuum). In these experiments, Giovannini et al. [56] dealt with single photons propagating in a confocal telescope. Two photons are generated as a photon pair that is strongly correlated in wavelength and generation time. The arrival times of the photons traveling through two different arms of an interferometer are compared with femtosecond precision using a quantum effect known as the Hong–Ou–Mandel effect. Then, they found that these arrival times indicate that photons did not travel at the same speed. G. Gouesbet met one of the authors, Miles Padgett, in March 2015, during a European/French Israeli Symposium on non-linear and quantum optics. Padgett expressed his disappointment that the news was badly received.

Actually, it has a very easy classical explanation drawn in Figure 3 which is quite similar to Figure 2. Photons travel at the speed of light in the direction of propagation of the tilted waves which is not the direction of propagation of the beam. The velocity V of a photon in the direction of propagation of the beam is actually equal to c cos θ in which θ is the axicon angle of a tilted plane wave. An interesting case is the one of Bessel beams whose tilted waves all have the same tilt θ, which is the axicon angle of the whole beam. Then, we determined that the speed of a laser light of Bessel beams can be made very slow, actually in principle arbitrary slow (notwithstanding the fact that there would be experimental practical limitations and that Bessel beams are not physical because they propagate an infinite amount of energy). A quantitative analysis of the effects shown in Figures 2, 3 is available in the work of Gouesbet and Lock [10].

FIGURE 3. Speed of laser light is slower than the speed of light.

3 Worldwide contributions to the failures of the optical theorem in electromagnetism

In this section, we deal with worldwide contributions toward the failures of the optical theorem, therefore restricting ourselves to the case of arbitrary-shaped beams and excluding generalized optical theorems under plane-wave excitation. We also exclude papers which, although dealing with non-plane excitation, deal with formulations that cannot be embedded in a framework using BSCs, excepted possibly if a failure caused due to non-plane-wave excitation is explicitly considered. Non-exhaustive examples of such papers which, for such reasons, are not deeply analyzed are by Jones [57], Carney et al. [53, 54], Gulyaev et al. [58], Lytle II et al. [55], Berg et al. [59, 60], Cotanch [61], Small et al. [62], Eremin and Svesnikov [63-65], Eremin [66]. A story of the issue is due to Newton [67]. There are also papers dealing with incident spherical waves emitted by point sources, such as the work of Athanasiadis et al. [68], leading particularly to a mixed reciprocity theorem which relates plane-wave incidence to point-source incidence. This paper proposed by Athanasiadis et al. is quoted in [69] which dealt with the fact that the spontaneous emission from an emitter depends on the surrounding medium and the modification of the optical theorem in the case of incident point-source fields was examined. Point sources were also discussed by Eremin and Sveshnikov [63] who provided a version of the optical theorem for the field of a point source in the presence of a transparent semi-space. The case of invariant beams (another name for “non-diffracting” beams) was analyzed by Rondon-Ojeda and Soto-Eguibar [70] using an angular spectrum representation, ASR (which could be converted to an analysis in terms of BSCs, as observed in [51]) with applications to Rayleigh scattering and to Bessel beam scattering, as shown by Rondon and Lee [71], for a similar complementary discussion. Another paper by Rondon [72] proposed a general expression for the optical theorem in terms of localized waves (which have frozen waves as a special case), e.g., [73–76] and references therein. They used ASR to write a physical condition related to the optical theorem. Then, the author commented that he explored several general mathematical representations of generating structured beams and found that the use of localized waves were the best one to fulfill the physical solution, i.e., he missed the possibility to use BSCs. Although devoted to plane waves and outside of the main stream of the present review, it is worth quoting a paper proposed in [77] which dealt with the optical theorem in the case of weakly absorbing media, i.e., when the host medium surrounding scatterers is not transparent.

An issue correlated with the one of the optical theorem concerns the properties of diffracted light. Indeed, for a sufficiently large size parameter (ka ≫ 1) and small scattering angle (θ < 6°) and for a uniform plane wave, it is known that diffraction leads to a narrow and intense lobe in the forward direction, as stated by van de Hulst [2], that is to say in the same direction than the one used for the optical theorem. The same argument which has been used for the optical theorem, relying on Figure 2, may then be used for diffraction as well, such that van de Hulst statement holds for each tilted plane wave of ASR but does not hold for the structured beam as a whole. Then, we may expect departures between GLMT and diffraction theory (DT) in the forward direction.

Although the aforementioned argument was not yet understood in 1990, the study in [78] devoted to the comparison between GLMT and DT demonstrated that such departures do exist. In this study, the far-field light scattered by a spherical particle illuminated by an on-axis Gaussian beam, with the BSCs evaluated by using a localized approximation, was considered. The expression for the localized approximation is more general than the one in Eq. 12 because it incorporates the parameter z₀ which is the z-coordinate of the beam waist center with respect to the center of the scatterer, read as

where

which reduces to Eq. 12 when z₀ = 0.

Extensive comparisons between GLMT and DT for a scattering angle ranging from 0 to 0.04 radians (approximately 2°), both from transparent and opaque particles, with radii ranging from approximately 10 μm to approximately 30 μm, for a wavelength equal to 632.8 nm, then showed that this is indeed the case, particularly when the particle is not located at the beam waist center of the beam. Departures between GLMT and DT can be observed in the strict forward direction, i.e., for θ = 0°.

Another comparison between GLMT and DT is available in [79] where the sensitivity of the forward field to the particle axial position and beam width was studied for the case of an on-axis Gaussian beam both theoretically and experimentally, revealing significant departures from plane-wave scattering behavior. In particular, Figure 4 in [79] shows and compares a diffraction-based description of the forward field with GLMT simulations. Computations are carried out in the far-field referring to a criterion for the validity of the far-field approximation obtained as πa²/(λr) ≤ 0.2 [80] in which r is the distance from the center of the scatterer. The authors comment that the forward direction θ = 0 has a particular significance because the total intensity in the forward direction correlates with the extinction of the incident beam. It is then found that for w₀/a ≥ 1, i.e., when the particle interacts with what is locally a plane wave, from the point of view of the particle, GLMT and DT agree in contrast with the case w₀/a ≤ 1, when the beam is essentially “blocked” by the particle, in which the diffraction-based description cannot completely account for the behavior of the total field in the forward direction. Another interesting result concerns the efficiency factor Q_ext defined as the scattered power divided by the power that is geometrically incident upon the particle, the latter being evaluated accounting for the fact that the incident beam is not a plane wave, leading to

from which we may obtain the usual plane-wave result Q_ext = C_ext/(πa²) when w₀ → ∞. Then, Figure 3 of [79] shows that Q_ext rapidly tends to become 2 when the particle radius is large enough. This is another justification of an assumption made by Lock [24] when studying the wide-beam approximation.

A discussion of extinction in Gaussian beam scattering by Lock has been available in the same year [81]. This paper is not related to the optical theorem so far as it does not focus on the forward direction nor on comparisons with the plane-wave behavior, but it is worth being quoted in the present review paper because it provides a thorough analysis of the behavior of extinction (more specifically of extinction efficiency Q_ext) in the case of on-axis arbitrary-shaped beam illumination (more specifically the Gaussian beam). Two expectations are discussed when the particle is non-absorbing. For the first one, it is expected that in the large-particle limit a ≫ λ, that is to say when the particle is illuminated by a wave somehow similar to a plane wave, Q_ext is approximately equal to 2.0 (a result already mentioned above), half of this value being due to the diffraction of rays that graze the edge of the particle, the other half being understood as the deflection of the geometrical rays that strike the particle surface. The second expectation concerns the case when a narrow beam is incident upon a large particle. The portion of the wave that grazes the particle’s edge is exceedingly weak, so the contribution of diffraction should be 0, and Q_ext should be equal to 1. The first expectation is confirmed by numerical computations, while the second expectation is not confirmed. Instead, in the narrow-beam limit, Q_ext as a function of the particle size parameter continues to oscillate approximately about 2.0. These features are examined in the GLMT framework using the localized approximation of Eq. 12, with Q_ext defined by an equation similar to that of Eq. 33, although the denominator used is a bit more complicated. This study conducted by Lock must be complemented by the paper proposed by Lai et al. dealing with the case of a two-dimensional light beam interacting with a long transverse cylinder without absorption, assuming short wavelengths, i.e., large size parameters ka, where a is the radius of the cylinder cross section [82].

In [83], a small particle is located in front of a glass prism in which a plane wave is propagating with incidence at a subcritical angle. This plane wave at the surface of the prism is partly reflected and partly refracted. The refracted wave may be expressed using either a p- or an s-polarization wave. Expressions for the scattering and extinction cross sections have been derived, assuming that the illuminating wave is an evanescent wave, using a formulation that is somewhat similar to GLMT formalism, i.e., relying on the expansion of fields (incident, scattered, and in the interior of the sphere) and on the use of boundary conditions. The definition of cross sections for evanescent-wave excitation allows quantitative comparison with the case of plane-wave excitation. It is found that, within the dipole approximation, the cross sections for plane-wave excitation lies between those for p- and s-polarized evanescent-wave excitation. It is also found that higher multipole contributions are strongly enhanced as compared to plane-wave excitation. It is mentioned that the present approach has already been discussed in the case of Gaussian beam illumination, referring to papers mentioned previously in the present review [24, 31, 81]. Numerical results are explicitly provided in the case of scattering of evanescent waves by “small” silver particles, such as for particles with radii equal to 5 and 100 nm and wavelengths between 300 and 1,000 nm.

Returning to the optical theorem, we now have a paper by Mitri [84] that dealt with the generalization of the optical theorem in cylindrical coordinates when an arbitrary EM-shaped beam illuminates an elongated object of arbitrary shape. The theoretical analysis relies on a GLMT-like approach, in which the fields are expanded in terms of vector wave functions, and the relationship between incident and scattered waves are obtained through the use of boundary conditions, see [85] for a review. The incident beam is then encoded by using cylindrical BSCs A_n(k_z) and B_n(k_z) in which k_z is the axial wavenumber of the incident field. Similar to the double-quadrature method used in GLMT for spherical particles [86], the cylindrical BSCs are expressed by a double-quadrature (over the scattering angle and over the axial coordinate). Asymptotic limits for the cylindrical Bessel and Hankel functions and their derivatives are considered in the far-field, although this is not compulsory as discussed in Appendix. The main result is then provided by Eq. 16, which is given with a slight modification, according to

where k_r is the radial wavenumber and a_n and b_n are scattering coefficients of the objects determined by applying boundary conditions, similar to the Mie coefficients a_n and b_n in spherical coordinates. The constant K, homogeneous to a length, may be viewed as a normalization constant, which is considered to be the length L of the cylinder in [84], which may also be considered to be 1, or better defined according to the actual properties of the incident beam.

Section 2 deals with on-axis beams, where BSCs were uni-index coefficients. However, in general, they are double-index coefficients, traditionally denoted as $g_{n,TM}^m$ and $g_{n,TE}^m$ (n from 1 to ∞, m from (−n) to (+n), TM for “transverse magnetic,” and TE for “transverse electric”), e.g., [5]. In cylindrical coordinates, BSCs depend on two indices, but one (n) is discrete, while the other (k_z) is continuous. Accordingly, C_ext of Eq. 34 is expressed by a summation associated to n and with an integral associated with k_z. This contrasts with the spherical case where, BSCs having two discrete indices, the spherical C_ext is expressed by a double summation. Finally, let us note that although [84] provides a generalized optical theorem, there is no explicit comparison with the case of a plane-wave incidence so that the failure of the optical theorem is not explicitly described, a possible issue for future work.

Then, the work in [87] experimentally demonstrated a complete violation of the classical optical theorem in the case of radially polarized beams at both visible and microwave frequencies. It was shown that a plasmonic particle illuminated by such a beam experiences a strong extinction in contrast with the fact that they have 0 scattering in the forward direction. They argued that the violation is a direct consequence of the appearance of the longitudinal field components at the focal spot where the scattering object is located. They also provided a generalized optical theorem which provides a good agreement with the observed results. Similarly to the study proposed by Rondon-Ojeda and Soto-Eguibar [70], their generalized theorem is established by relying on ASR (and is not expressed in terms of BSCs). Numerical calculations are carried out by using finite element software dealing with the scattering from a 100 nm spherical gold nanoparticle and comparing linearly and radially polarized beam field distribution obtained in the paraxial approximation. Experiments are carried out with optical Fourier microcopy with 100 nm gold colloidal nanoparticles and microwave scanning microscopy at the frequency of 9.5 GHz with a 3.5 mm sphere made from stainless steel.

This section is concluded with a review paper by Markel, dealing with the extinction of electromagnetic waves [11]. The optical theorem is specifically discussed in Section 4.4 in [11]. The case of a single plane wave is discussed in Section 4.4.1. The case of several plane waves is considered in Section 4.4.2. This case is particularly interesting in the present paper because it matches the explanation of the failure of the optical theorem shown in Figure 2, although the author dealt with a discrete superposition of plane waves, in contrast with the fact that the ASR of an arbitrary-shaped beam, in general, is a continuous spectrum. The section then proposes a generalized optical theorem for several incident plane waves oscillating at the same frequency, in which Q_ext is expressed by a discrete summation. The author succeeds to reach the gist of the failure by noting that, “in the case of several incident plane waves, there is no well-defined forward direction, and therefore, the extinction cross section does not have the same simple interpretation as in the case of a single plane wave,” an explanation already available in [10] for the more general case of arbitrary-shaped beams (laser beams in practice).

4 Generalized and extended optical theorems in acoustics and applications

Similar to the case of electromagnetism, failures of a classical optical theorem in acoustics lead to an extended optical theorem in acoustics which is reviewed in the present section.

4.1 Some applications of the regular optical theorem in acoustics

To appreciate the context of subsequent developments, it is appropriate to recognize selected applications with plane-wave illumination up to the year 2000. Morse and Feshbach in their 1953 textbook [88] included an analysis of the extinction cross section of a sphere illuminated by a plane wave along with a discussion of the absorption and scattering cross sections. The extinction is equivalent to the ordinary optical theorem, Eq. 3, by combining equations given on pages 1488 and 1489. In the more specialized 1968 textbook devoted to acoustics, Morse and Ingard [89] on pp. 426 and 427 explicitly expressed the extinction power in terms of the complex forward scattering amplitude as here in Eq. 3. During that era, an appreciable fraction of researchers interested in acoustical scattering had completed the coursework in quantum mechanics, and it was unnecessary to emphasize the relationship with quantum mechanical scattering theory and the generalization with appropriate notation to three-dimensional acoustical scattering theory by non-spherical objects. Expressions relating the energy flux density to the local acoustic amplitudes can be found in the publications referenced in this section. In many acoustical publications, cross sections are designated by σ instead of by the symbol C used in the present article.

By 1990, Kargl and Marston [90] used results equivalent to Eq. 3 to compute the extinction of sound by an empty elastic shell in water in the absence of significant energy absorption. The complex scattering amplitude was evaluated using a partial-wave series which was accurate for the idealized case in which there was no absorption of energy in water. Using a notation analogous to Eq. 3, the normalized cross section C_ext/(πa²) (where a is the shell’s outer radius) was plotted as a function of ka, where k = 2π/λ and λ is the wavelength in water. The important result is that C_ext/(πa²) differed significantly from the analogous result for a rigid sphere in water throughout the range ka of 0–100. For ka as large as 100, C_ext/(πa²) for the shell differed significantly from the usual extinction paradox value of 2, and it displayed a supposed structure approximately periodic in ka. That additional structure was modeled using quantitative ray theory that determined the contribution to forward scattering caused by elastic waves guided by the surface of the sphere. The elastic waves are excited by the illumination in regions offset from the associated symmetry axis such that the strength of the forward scattering is enhanced by a form of glory scattering accounted for in the ray theory. It is noteworthy that the measurements of the forward scattering amplitude using sufficiently short duration tone-burst illumination revealed that guided wave contributions arrived at the hydrophone before the ordinary forward diffraction contribution associated with diffraction in the region of the sphere’s equator. That is the expected relative timing because in the ka region of interest, the group velocity of the guided elastic waves significantly exceeds the speed of sound in water. See the ray diagram in Figure 2 and the data in Figure 9 of [90]. It is evident that surface-guided elastic waves and associated resonances complicate the interpretation of C_ext/(πa²) for elastic shells in water even when ka is as large as 100. For each resonance, there is an associated partial-wave contribution.

4.2 Acoustic generalized optical theorem (GOT) with plane-wave illumination

A derivation of GOT for a broad class of non-absorbing scatterers producing three-dimensional scattered waves was published in 2001 [91]. The GOT terminology used in the present section follows that in the final edition (1968) of Leonard Schiff’s acclaimed textbook [92]. The author primarily responsible for the present section, Marston, can recall the profound impact of Professor Schiff on experimental as well as theoretical physics research at Stanford University, and the priority provided to his terminology is appropriate in the present context. To present the acoustical case [91], let p_i denote the incident pressure amplitude phase referenced to a point at the center of the symmetry of the scatterer and let r be the distance from that point to the far-field observer. The far-field complex scattered pressure is expressed as follows using the exp(−iωt) time convention (which is not explicitly expressed here and in subsequent developments, and opposite to the usual exp(iωt) convention used in GLMT):

where n_i and n are unit vectors in the direction of the incident and scattered waves. The complex scattering amplitude A is a function of the size and shape of the scatterer, its material properties, and k = ω/c, where c is the speed of sound in the surrounding fluid. In the GOT discussed here, the dissipation of energy in the surroundings and in the scatterer are neglected such that the absorption cross section vanishes. Under these circumstances, reciprocity holds giving A(n,n_i) = A(−n_i,−n), corresponding to a reversal of the relative directions. In addition, it is convenient to make the following assumption concerning the shape and material properties of the scatterer: inversion symmetry is satisfied by the scatterer. Consequently, there is no change in the properties of the scatterer when r is replaced by −r, where r is a vector from the center of symmetry. It follows that A(n,n_i) = A(−n,−n_i). Letting Im denote the imaginary part of a complex quantity and * denote complex conjugation, the result of the analysis, Eq. 13 of [91], is as follows:

where the solid angle differential dΩ pertains to the scattered direction n within the integral and the integration is over 4π steradians. The theorem allows a component of the complex scattering amplitude in an arbitrary direction n′ to be expressed in terms of an angular integration involving scattering amplitudes evaluated at different directions n. The usual optical theorem for the plane-wave illumination of a dissipationless target is given by taking n′ = n_i giving 4πIm[A(n_i,n_i)] = kC_sca, which is equivalent to Eq. 3 for this situation. With n′ = −n_i, A(n′, n_i) becomes the backscattering amplitude and that special case of GOT was confirmed using numerical integration for a perfectly soft sphere in [91]. For the special case of scattering by spheres, A(n, n_i) can be expressed using a partial-wave series with coefficients in the series expressed as proportional to (1 − s_n) for the nth term [91]. The convention has been widely used in acoustical scattering theory since the 1970s and has the advantage that the complex s_n are such that $\left|s_n\right|=1$ in the absence of dissipation. Furthermore, that convention simplifies the comparison with standard results from quantum mechanical scattering theory and will be retained in the present section. The reader is cautioned that some recent authors designate a partial-wave quantity s_n in a different way occasionally resulting in erroneous expressions for acoustical results. Equation 36 was also shown to be relevant to certain multiple scattering problems [91]. The acoustic GOT in Eq. 36 is relevant to a combination of analytical approaches to scattering and imaging [93].

4.3 Unusual scattering and radiation force properties of spheres on the axis of zero-order acoustic Bessel beams

To appreciate the need for an acoustical extended optical theorem (EOT), it is appropriate to review the predicted scattering and radiation force properties of spheres placed on the axis of the acoustical Bessel beams. While it has been noted previously that a true Bessel beam transports an infinite amount of power, the power transported by a plane wave of infinite extent also diverges and the scattering theory for both idealized cases are worthy of attention. The complex scalar wave zero-order Bessel function beam solution of the Helmholtz equation is

where $R={(x^2+y^2)}^{1/2}$ is the radial coordinate in a cylindrical sense, z is the axial coordinate, μ² + κ² = k² = (ω/c)², μ is non-negative, and κ is conveniently expressed in terms of the conic angle β as κ = k cos β. While some of the modern interest in invariant beams of this type and the associated geometric interpretation involving the angle β were stimulated by Durnin [94], the explicit geometric interpretation goes back to as far as lectures conducted by Schelkunoff on waveguide modes in 1942 [95], where Bessel wave fields were expressed using a “cone of directions of elementary uniform plane waves.” The constant ψ₀ expresses the magnitude and in some cases a phase constant of the wave. In the earliest publication on the scattering by spheres in acoustic Bessel beams, ψ_B0 was considered to be an acoustic velocity potential ψ related to the local complex velocity and acoustic pressure by u = ▽ ψ and p = iωρ₀ψ, where ρ₀ is the density of the surrounding fluid. It is to be noted that for a given ω, p is proportional to ψ. The general solution for the far-field scattering for a sphere of radius a centered at z = 0 on the axis of the beam is ψ_s = (a/2r)ψ₀f_B0 exp(ikr), where the dimensionless form function f_B0 is [96–99]

where P_n are Legendre polynomials, θ is the scattering angle relative to the z-axis, and the complex s_n depend on ka and on the material properties of the sphere and of the surrounding fluid, but not on the beam parameter β (Figure 1 of [96] shows the parameter β and the sphere). When β = 0, P_n(cos β) = 1, and the usual scattering solution for plane waves incident on the sphere is recovered. The s_n are known for many types of spheres and in the special case of no absorption of power $\left|s_n\right|=1$, as previously noted. An inspection of Eq. 38 shows that the contribution of specific partial waves to the scattering can be suppressed by selecting β such that P_n(cos β) = 0 [98]. This observation served to motivate the needed extension of the optical theorem.

Another justification for considering an extended optical theorem is the effect of β on the axial component of the radiation force F_rad on spheres. By 2006, conditions were found where F_rad < 0 corresponding to what is known as a tractor beam [96, 99]. In all cases where F_rad < 0, the magnitude of the scattering into the backward hemisphere was suppressed. In agreement with those observations, in 2011, F_rad was expressed directly in terms of the extinction power P_ext and the asymmetry of the scattering $⟨\cos \theta ⟩$ [100]as follows:

where the scattered power P_sca is proportional to the denominator of the scattering asymmetry

where w = cos θ and f_B0 (w) is given by Eq. 38 for the present case of zero-order Bessel beam illumination. Some readers may find the related “open-access” discussion in [101] helpful.

The dependence on P_ext motivated the interest in an extended optical theorem even though other ways of evaluating P_ext and F_rad were available in [98]. The result in Eq. 39 provides a simple interpretation of negative F_rad associated with momentum transfer [100–102]. Figure 1 of [102] shows a helpful momentum transfer diagram. By 2011, a corresponding result for light scattering had appeared [103]. By 2012, the result in [92] had been generalized to arbitrary scatterers in arbitrary diffraction-free beams [101] and a partially analogous optical tractor beam had been demonstrated [104]. By 2014, an acoustical tractor beam was demonstrated for a specialized target having a large scattering asymmetry [105].

4.4 Simplest extended optical theorem example—an axisymmetric object on the axis of a zero-order Bessel beam

While the acoustical EOT derived in [101] and discussed in [106] is more general, since those publications (and a related one in [107]) are “open access,” for the purpose of illustration, the simplest example will be shown here which is zero-order Bessel beam illumination. Some readers may find the diagram in Figure 1 of [106]helpful. Let the scattering amplitude be denoted by ψ_s = ψ₀[A_s(θ, ϕ)/r] exp(ikr), where ϕ is the azimuthal angle and ψ₀ is defined as in Eq. 37. For the present axisymmetric case, the EOT in Eq. 9 of [101] reduces to an angular integration over the angular spectrum of the illumination, giving the extinction cross section as

where β is the conic angle of the beam. Equation 41 further reduces this situation to

since for the present case, A_s does not depend on the azimuthal angle ϕ. When the conic parameter β = 0, corresponding to plane-wave illumination, Eq. 42 reduces to the usual optical theorem [107]. For the present example, the EOT in Eq. 42 necessitates the evaluation of A_s at the conic angle β of the Bessel beam. The correctness of this result is easily verified for scattering by a sphere where an independently derived result for C_ext is available [98]. In that case, A_s = (a/2)f_B0, where f_B0 is given by Eq. 38, so Eq. 42 reduces to

where Re denotes the real part. Equation 43 agrees with the direct analysis from conservation laws in Eqs 16–18 of [100]. When β = 0, P_n(cos β) = 1 for all n and Eq. 43 reduces to the usual optical theorem. It is to be noted that the nth partial-wave contribution is suppressed by selecting β such that P_n(cos β) = 0 [98]. Consequently, with Bessel beam illumination, the effect of specific elastic modes on C_ext for spherical shells in water (previously noted in relation to [90]) can be suppressed.

Another method of verification is available by using Babinet’s principle, which is an approximation in the case of a fixed rigid disk having ka ≫ 1 placed on the axis of a Bessel beam. The resulting approximation for A_s(β, ϕ), which again does not depend on ϕ, may analytically be evaluated giving a simple approximation for C_ext. The result is compatible with the result of the partial-wave series for a fixed rigid sphere, when the appropriate projection of the disk area is included in the analysis [108].

4.5 Extension of EOT to acoustical vortex beams

Another example of non-plane-wave illumination is the case of integer-order acoustic vortex beams (VBs) with a complex velocity potential of the form

where ϕ is an azimuthal angle of the beam axis and ψ₀ and Z are separate magnitude and phase functions, respectively. An acoustic beam in water demonstrated near the axis to have the dependences in Eq. 44, in which m = 1 was demonstrated by 1998 using an appropriately phased array of source transducers [109]. A special case of such a beam is an idealized Bessel VB: ψ_BVB(R, z, ϕ) = ψ₀J_m(μR) exp(iκz + imϕ), where μ and κ are defined in Eq. 37. By 2006, the effect on the scattering of taking m = 1 was known [110], and soon thereafter, the radiation force F_rad was also known [111]. For the general integer m case and the associated geometrical interpretation, see [100] (a formal proof of the conic representation is in the appendix of [110]). The result of the EOT, Eq. 43 for m = 0, for spheres in the axis of an arbitrary integer-order Bessel beam is given by Eq. 12 of the open-access publication [106], which is equivalent to the direct calculation of extinction obtained in Eq. 17 of [100]. In the discussion which follows, it is noteworthy that in the partial-wave series expansion for extinction, absorption, and scattering cross sections, each contains a factor ${[P_n^m(\cos \beta )]}^2$, where $P_n^m$ is an associated Legendre function. The possibility of significantly modifying contributions associated with elastic resonances previously discussed for m = 0 is also present for the arbitrary integer m case.

In the case of axisymmetric objects placed on the axis of VBs, calculating the absorbed power P_abs has additional significance. By 1999 [109], it was realized that the axial radiation torque N on the object is N = mP_abs/ω though the method of derivation was limited to paraxial VBs. By 2011, the derivation had been extended to include non-paraxial VBs, the result having been published in the abstracted form in 2009 [112] (for comparison, from Maxwell’s equations, the radiation torque on a sphere illuminated by circularly polarized light is P_abs/ω [102, 113]). In the acoustic case, the prediction N = mP_abs/ω was observed in 2012 and supported by experiments [114]. For a sphere in a Bessel VB, the partial-wave expansion for P_abs [100] contains terms proportional to $(1-{\left|s_n\right|}^2){[P_n^m(\cos \beta )]}^2$. For spheres in certain size ranges, it becomes necessary to include the effects of viscous energy dissipation in the surrounding fluid in the evaluation of $\left|s_n\right|$ for the n = 1 (dipole) term [115]. When the fluid parameters are such that the viscous Stokes layer thickness is small in comparison with the particle radius, for dense particles with ka ≪ 1, it has been possible to numerically confirm (using finite elements) that the approximation in [115] is useful for predicting the radiation torque and, thus, P_abs for a wavefield having m = 1 [116].

It is noteworthy that for all types of illumination, P_abs is non-negative for all scatterers lacking internal energy sources, which are known as passive scatterers, the usual case of interest. Though this was noted in [101], by 2016, it was necessary to publish a detailed proof applicable to arbitrary source waves and geometries [117] as a consequence of assertions made to the contrary by other researchers. For the case of scattering by spheres, the analytical expression for P_ext, P_abs, P_sca, and F_rad may be converted to useful forms by defining δ_n and non-negative γ_n such that s_n = exp[2i(δ_n + iγ_n)], where δ_n + iγ_n is a complex partial-wave phase shift using terminology used for quantum mechanical scattering. The relevant expressions for plane-wave and mth-order Bessel beam illumination are derived in an open-access publication [118]. For examples of applications with γ_n = 0, see [119], which includes the case of Bessel standing waves, and [120] for the optimization of tractor beams.

4.6 Extinction for two-dimensional scattering and other research

By 2000, the close analogy between the ordinary acoustical theorem and the quantum mechanical three-dimensional case was such that it was unnecessary to separately develop a theorem for two-dimensional (2-d) scattering. The optical theorem in the quantum 2-d case was by then well known [121, 122]. The acoustical 2-d result for extinction was by then textbook material [123]. The resulting series expressions for extinction, absorption, and scattering are reviewed in [117] in the form that is easily verified to agree with the quantum results [121, 122]. An alternative expression is proposed by Mitri [124]. By 2001, a 2-d generalized optical theorem had been developed and numerically confirmed for the acoustical case [125]. Justification for separately considering 2-d scattering is that the investigations of 2-d situations are often useful when developing new scattering theory [126, 127].

4.7 Relationship between acoustical theorems and some modern optical theorems

In this subsection, the terminology from Section 4.2 is retained where generalized optical theorems are similar to the ones reviewed in Eq. 36, in agreement with Newton’s terminology [67]. Theorems of the type discussed in [54, 55] and in Sections 4.4, 4.5 for non-plane-wave illumination become extended optical theorems. The scalar-field approach discussed in [54], as well as the acoustical approaches reviewed here, account for the interference resulting from the different plane-wave components of the illumination and associated components of the scattering. However, there is a distinction in the method of derivation. Carney et al. [54] assumed the scatterer to be characterized by a “susceptibility” associated with a spatial variation of the compressibility of a fluid medium considering only the case of fluid scatterers; however, if there are spatial variations in the fluid density, the wave field differs from the form considered in [54] (compare Eq. (8.1.12) of the work of Morse and Ingard [89] with Eq. 2 of [54]). However, in many acoustic scattering situations of interest, not only does the density of the scatterer differs from the surroundings but also the scatterer may be a solid object. Hence, the approach commonly used in acoustics is to begin with known solutions for the scattering by a plane wave and built up solutions for more complicated types of acoustic illumination by superposition [96, 100, 101, 106, 107].

5 Failures of the optical theorem in quantum mechanics

In quantum mechanics, there is a well-known version of the optical theorem which is a landmark of basically all textbooks on the issue. This classical version relies on the fact that when dealing with the quantum mechanical scattering of a beam of projectiles by a single force center, the projectile wave function is standardly modeled by a plane wave since both the amplitude variation along a phase front and the phase front curvature again are negligible over the volume of a single target [128]. As noted by [24], scattering by a transversely localized beam is generally not important in quantum mechanical scattering, since the wave function of a high-energy projectile incident on a small target is well modeled by a plane wave. However, the need to model the transverse localization of the beam is important for single or multiple scattering of the projectile beam by a cluster of targets when the cluster size is comparable to or larger than the beam diameter. In this field, the approach to encode the incident beam is by using an infinite series of partial waves, as revisited in [129] and used below to reformulate the optical theorem, following [130].

Classically, when dealing with illuminating plane waves, the optical theorem is usually expressed as follows [131–133]:

where $C_{\mathit{ext}}^q$ (q standing for “quantum”) is the quantum extinction cross section, k is a quantum wavenumber, k the associated wave vector, and f_k(0) is the scattering angular function associated to a quantum radial potential taken in the forward direction, for a scattering angle θ = 0. The complete scattering angular function (independently of the scattering angle) is given as follows:

in which S_l’s are quantities (in general complex numbers) with a modulus smaller than 1 and $Y_l^0$’s are spherical harmonics with quantum number m equal to 0. Equation 46 may be rewritten as follows:

which may be viewed as the quantum optical theorem expressed in terms of partial waves. It may be worthwhile to note a structural analogy between Eqs 43, 47, particularly for β = 0 (when the Bessel beam is a plane wave). Furthermore, in both Eqs 43, 47, the partial-wave summation ranges from 0 to ∞, due to the fact that both acoustical and quantum scatterings are scalar scatterings, in contrast with the vectorial electromagnetic scattering where the partial-wave summation starts from 1, e.g., Eq. 1.

We now consider the interaction of a radial quantum potential with a beam pertaining to a class of beams, called quantum eigenarbitrary-shaped beams, defined by a Fourier transform read as follows [129]:

in which Ψ(r) is an incident (frozen) wave function, a(k) = a(k, θ_k, φ_k) is the angular spectrum (with $k=\left|\mathbf{k}\right|$ fixed), and dΩ_k = sin θ_kdθ_kdφ_k is an infinitesimal solid angle, with k, θ_k, and φ_k spherical coordinates in the wavenumber space. The wave function may be expanded over free spherical waves ${\phi }_{\mathit{klm}}^{(0)}(\mathbf{r})$ according to

in which the free spherical waves read as follows [131]:

where j_l(kr) are the spherical Bessel functions of the first kind and $Y_l^m$ are the spherical harmonics. The expansion coefficients a_klm may be called quantum beam shape coefficients (QBSCs) so far as they play a role similar to the BSC-encoding electromagnetic beams. Equation 49 shows that the QBSCs encode the quantum beam. By inversion, we then show that these QBSC can be evaluated as follows:

where the star denotes, as usual, a complex conjugation. Any choice of QBSCs produces an eigenarbitrary-shaped beam. Given a set of QBSCs, we may compute the spectrum a(k) by using the inverse of Eq. 51 and then obtain the wave function of Eq. 48. This being said, we may evaluate the associated quantum extinction cross section reading as follows [134]:

which is the generalization of the classical optical theorem of Eq. 47, both of these equations being valid for the elastic and inelastic cases as well. Using the QBSCs of plane waves [135], we then demonstrate that Eq. 47 is recovered from Eq. 52 as a special case. Furthermore, the publication [130] discusses other special cases, such as on-axis Gaussian eigenarbitrary-shaped beams and on-axis Bessel eigenarbitrary-shaped beams.

The analogy between the Helmholtz equation in acoustics and the spatial dependence of steady-state quantum wave function Ψ(r) is such that quantum Bessel-shaped beams analogous to the acoustic Bessel beam in Eq. 37 were discussed in Eq. 23 of [130] and what corresponds to a QBSC for the Bessel beam case was given in Eq. 25 of [130]. The result obtained is directly analogous to the acoustic result reviewed in Eq. 38 from [96, 97]. In this case, the quantum result in Eq. 52 and Eq. 12 of [130] becomes analogous to the acoustic Bessel result here in Eq. 43 based on the extended optical theorem stated in [101, 106].

It is noteworthy in this context that quantum mechanical “matter-wave” Bessel tractor beams have been proposed [136] having a wave function analogous to Eq. 37 and scattering properties directly analogous to those considered in the original tractor beam case [96]. Unfortunately, the authors of [136] appear to have been unaware of research subsequent to [96], greatly increasing the magnitude of possible acoustic negative radiation forces on spheres as known in 2016 in [99, 100, 111]. Even larger acoustic negative force magnitudes for spheres and related issues were subsequently discussed, for example, in [119, 120, 137], [138, 139]. Perhaps the greater interest in the present review is that the expression for the stationary force on a scatterer centered in a matter-wave Bessel beam, Eq. 4 of [136], is expressed using quantum mechanical partial-wave phase shifts analogous to the acoustic phase shifts δ_n discussed in Section 4.5. Furthermore, the expression relating to the force and the δ_n in the quantum Bessel beam case, has the same form as the acoustic result in Eq. 26 of the open-access publication [118]. The result in [118] is actually more general in that it also applies to acoustic Bessel vortex beams. For other examples of how acoustics provides useful expressions for scattering and radiation forces partially analogous to quantum mechanical expressions, see [140].

6 Conclusion

Advances in science regularly occur when apparently well-established results are found to be obsolete. One such well-established result is the optical theorem. It was a major surprise when G. Gouesbet discovered in the early 1980s that this theorem was not valid for structured beams. More surprisingly, the deep understanding of the reason for the failure of the classical optical theorem had to wait more than 30 years, and still more surprising is the fact that this reason could be explained with plain words in a very easy way. Since then, several papers dealt with the same issue and produced generalized (or extended) optical theorems, not only in electromagnetism but also in acoustic and quantum mechanics. This paper presented a comprehensive review of the literature devoted to the optical theorem when the scatterer is illuminated by structured beams.

Author contributions

GG: writing–original draft. PM: writing–review and editing.

Funding

The Washington State University portion of this research by PM was funded in part by the US Office of Naval Research under award number N000142212599.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References